Parameter Identification on Time-Dependent Domains using Adaptive Finite Cell Methods
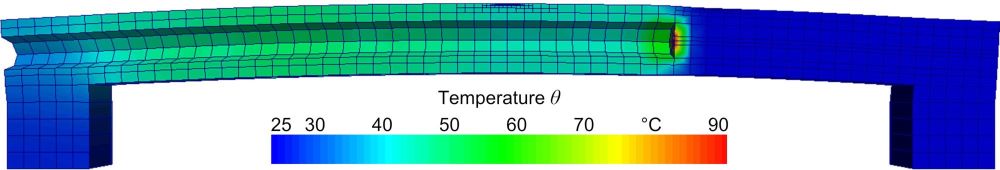
Motivated by an industrial deep hole drilling process, we consider partial differential equations on time-dependent domains. While elastic deformations due to applied forces and induced heat can be represented by thermoelastic material models, the time-dependent material removal leads to a reduction of the workpiece. The simulation of such processes with a detailed inclusion of the material removal is extremely computationally expensive and complicated. Therefore, we use a simpler substitute model that relies on parameters which cannot be determined directly by measurements. Instead, they are identified numerically. The time dependence of the domain is treated by the so-called finite cell method (FCM). It combines the usual space-time finite element discretisation with a fictitious domain approach, involving a special numerical quadrature to treat the arising discontinuous integrands. The described procedure contains some error sources that affect the accuracy of the numerical solution: fictitious domain errors, space- and time discretisation errors, integration errors and numerical errors. Therefore, the dual weighted residuals (DWR) method is used to estimate the individual error components a posteriori. Based on this, an adaptive strategy is developed that balances the mentioned error components. In summary, this project aims for an adaptive method for parameter identification in parabolic problems with underlying time-dependent domains.
 Motivated by an industrial deep hole drilling process, we consider partial differential equations on time-dependent domains. While elastic deformations due to applied forces and induced heat can be represented by thermoelastic material models, the time-dependent material removal leads to a reduction of the workpiece. The simulation of such processes with a detailed inclusion of the material removal is extremely computationally expensive and complicated. Therefore, we use a simpler substitute model that relies on parameters which cannot be determined directly by measurements. Instead, they are identified numerically. The time dependence of the domain is treated by the so-called finite cell method (FCM). It combines the usual space-time finite element discretisation with a fictitious domain approach, involving a special numerical quadrature to treat the arising discontinuous integrands. The described procedure contains some error sources that affect the accuracy of the numerical solution: fictitious domain errors, space- and time discretisation errors, integration errors and numerical errors. Therefore, the dual weighted residuals (DWR) method is used to estimate the individual error components a posteriori. Based on this, an adaptive strategy is developed that balances the mentioned error components. In summary, this project aims for an adaptive method for parameter identification in parabolic problems with underlying time-dependent domains.
Motivated by an industrial deep hole drilling process, we consider partial differential equations on time-dependent domains. While elastic deformations due to applied forces and induced heat can be represented by thermoelastic material models, the time-dependent material removal leads to a reduction of the workpiece. The simulation of such processes with a detailed inclusion of the material removal is extremely computationally expensive and complicated. Therefore, we use a simpler substitute model that relies on parameters which cannot be determined directly by measurements. Instead, they are identified numerically. The time dependence of the domain is treated by the so-called finite cell method (FCM). It combines the usual space-time finite element discretisation with a fictitious domain approach, involving a special numerical quadrature to treat the arising discontinuous integrands. The described procedure contains some error sources that affect the accuracy of the numerical solution: fictitious domain errors, space- and time discretisation errors, integration errors and numerical errors. Therefore, the dual weighted residuals (DWR) method is used to estimate the individual error components a posteriori. Based on this, an adaptive strategy is developed that balances the mentioned error components. In summary, this project aims for an adaptive method for parameter identification in parabolic problems with underlying time-dependent domains.

